19 Jul On divisibility of the solutions of Pell’s equation
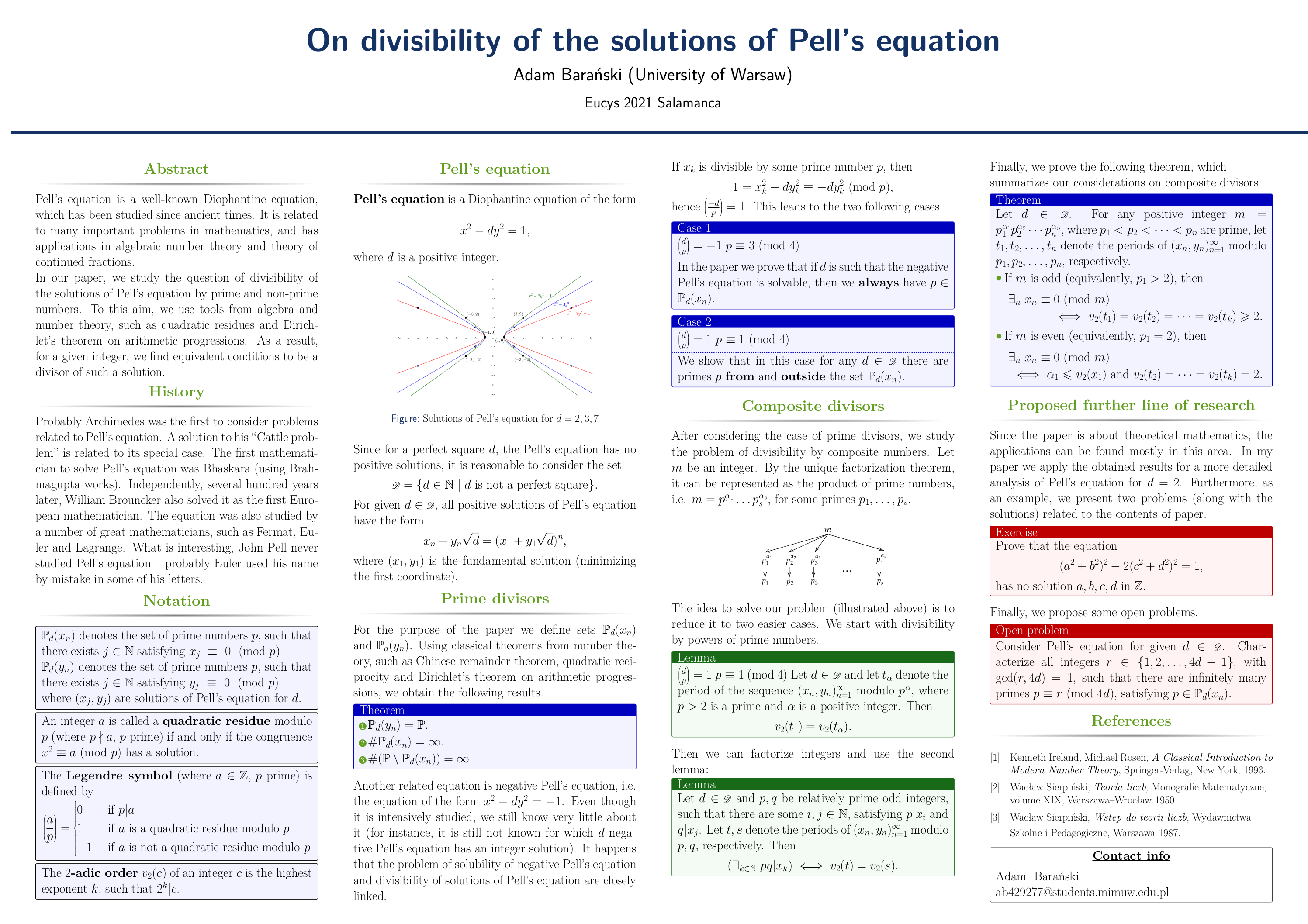
Pell’s equation is a well-known Diophantine equation, which has been studied since ancient times -first by the Greek (the famous cattle problem of Archimedes) and Indian mathematicians (Bhaskara, Brahmagupta), and then in Europe (Brouncker, Euler, Fermat, Lagrange). Pell’s equation is related to many important problems in mathematics, and has applications mainly in algebraic number theory and theory of continued fractions. In my paper, I study the question of divisibility of the solutions of Pell’s equation by prime and non-prime numbers. To this aim, I use tools from algebra and number theory, such as quadratic residues and Dirichlet’s theorem on arithmetic progressions. As a result, for a given integer, I find equivalent conditions to be a divisor of such a solution.
Category: MATHEMATICS — Country: POLAND — Year: 2020

Adam Stanisław Barański